Chromatic Derivatives and Applications
CHROMATIC DERIVATIVES AND APPLICATIONS
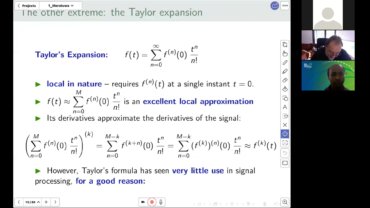
Chromatic derivatives are special, numerically robust differential operators which preserve spectral features of a signal; the associated chromatic expansions, when truncated, is similar to Taylor series expansion, however, they capture more spectral information, such that it gives better local approximations which accurately capture local features of a signal than the traditionally used Taylor series or Shannon expansion. This allows digital processing of continuous-time signals often superior to processing of discrete samples of such signals.
This online webinar commenced with a welcome and introduction about the speaker, Prof. Aleksandar Ignjatovic, given by the Staff Advisor of the IEEE SPS Student Branch Chapter of University of Moratuwa. The motivation for introducing these notions and their main properties were explained in a very comprehensible manner. A few applications of this remarkable invention were also discussed at the end of the session. The audience was benefitted with a 30-minute Q&A session to discuss any queries on the subject matter. This interesting session concluded with the Vote of Thanks by the Secretary of the society.
About the speaker
University of New South Wales, Australia
Biography:
Prof. Aleksandar Ignjatovic received the PhD degree in mathematical logic from the University of California, Berkeley. He is an Associate Professor in the School of Computer Science and Engineering at the University of New South Wales, Australia. His current research interests include approximation theory, sampling theory and applied harmonic analysis, algorithm design and applications of mathematical logic to computational complexity theory.